Unraveling the Mystery of Undefined Terms: The Foundation of Geometry
Imagine trying to build a skyscraper without any concrete or steel, just thin air. Sounds impossible, right? Well, that's what it's like to approach geometry without understanding undefined terms. They're the invisible foundation upon which the entire world of shapes, sizes, and spatial relationships is built.
We toss around words like "point," "line," and "plane" in geometry class as if they're as tangible as a ruler or protractor. But have you ever stopped to really think about what these words truly mean? Can you precisely define a "point" without resorting to other geometric terms? It's tougher than it seems!
This is where the fascinating concept of "undefined terms" comes into play. In geometry, we accept certain fundamental concepts as building blocks without providing rigid definitions. Instead of getting bogged down trying to define the undefinable, we use these undefined terms as the starting point, the bedrock upon which we construct all other geometric definitions, theorems, and proofs.
Think of it like a game. Every game has its rules, and those rules rely on some basic elements that everyone agrees on before they start playing. You can't explain chess without first establishing what a pawn, a rook, and a king are. Similarly, geometry has its "game pieces" – undefined terms – that are the foundation for everything else.
So, if you're ready to dive into the world of angles, shapes, and proofs, buckle up! Understanding undefined terms is your ticket to unlocking the elegant logic and surprising beauty of geometry. Let's explore why these seemingly simple concepts are so crucial to understanding the very fabric of space itself.
Now, let's dive into these crucial undefined terms: point, line, and plane.
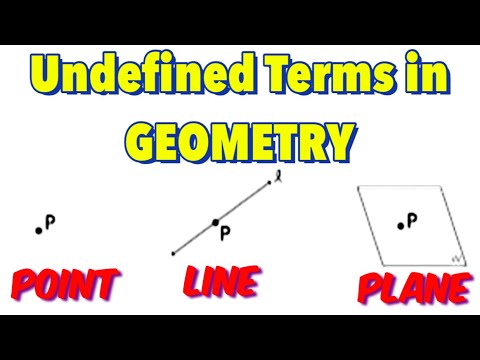
Point: Imagine the tiniest dot possible, so small it takes up virtually no space. That's the essence of a point. It has no size or dimension, just location. We represent it with a dot and a capital letter, like point A.
Line: Picture a perfectly straight path that extends infinitely in both directions. That's a line. It has no thickness, only length. We represent it by drawing a line with arrows at both ends and naming it with a lowercase letter or two points on the line, like line l or line AB.
Plane: Think of a flat surface that extends infinitely in all directions. That's a plane. It has length and width but no thickness. We represent it with a slanted four-sided figure and name it with a capital letter, like plane P.
While we don't formally define these terms, we use them to define everything else in geometry. For example, a line segment is defined as the part of a line that lies between two points. See how undefined terms act as the building blocks?
So, why is this important? Understanding undefined terms helps us:
1. Build a Solid Foundation: Just as a house needs a strong foundation, geometry needs clear starting points. Undefined terms provide this foundation, preventing circular definitions and ensuring everything is built on solid ground.
2. Develop Logical Reasoning: Geometry is all about logical thinking and proof. By accepting undefined terms, we can build a chain of reasoning that leads to powerful theorems and insights.
3. Connect to the Real World: While points, lines, and planes are abstract concepts, they help us model and understand the physical world around us. From architecture to computer graphics, undefined terms are the basis for many practical applications.
Although we don't have a set of steps or a checklist for using undefined terms, the key is to internalize their meaning. When you encounter them in definitions or theorems, take a moment to visualize their essence. Think of them as the fundamental particles that make up the language of geometry.
Advantages and Disadvantages of Undefined Terms in Geometry
While not typically framed as having advantages and disadvantages, let's explore the strengths and potential limitations of using undefined terms:
| Advantages | Potential Limitations |
|---|---|
| Provide a clear and unambiguous starting point for geometric reasoning. | Can be initially abstract and challenging to grasp for learners accustomed to concrete definitions. |
| Avoid circular definitions and ensure the logical consistency of geometry. | May require a shift in thinking to embrace concepts that are not explicitly defined. |
Here are some best practices for incorporating undefined terms into your study of geometry:
1. Embrace the Abstract: Don't get hung up on trying to find concrete definitions. Accept that points, lines, and planes are abstract concepts that represent the foundation of geometry.
2. Visualize and Draw: Use diagrams and drawings to represent undefined terms and the geometric figures built upon them. This can help make abstract concepts more concrete and easier to understand.
3. Connect to Definitions: Pay close attention to how undefined terms are used in the definitions of other geometric terms. This will deepen your understanding of how they serve as building blocks for more complex concepts.
4. Apply to Real-World Examples: Look for examples of points, lines, and planes in the world around you. This can help you make connections between abstract concepts and real-world applications.
5. Practice, Practice, Practice: The more you work with undefined terms and geometric concepts, the more intuitive they will become. Solve problems, prove theorems, and engage in geometric reasoning to solidify your understanding.
Here are some common questions and answers about undefined terms in geometry:
Q1: If we don't define them, how can we use them?
A1: We use them as starting points, like basic rules everyone agrees on. Their meaning comes from how they relate to other terms and are used to build more complex ideas.
Q2: Are there other undefined terms in geometry?
A2: Yes, while point, line, and plane are the most common, other undefined terms like "set" and "betweenness" also play a role in building the foundations of geometry.
By understanding undefined terms, we unlock the gateway to a world of geometric discovery and application. From the intricate proofs of Euclidean geometry to the practical applications in fields like architecture, engineering, and computer science, these fundamental concepts are the silent heroes behind the scenes. So, the next time you encounter a "point" or a "line," remember that you're looking at the very building blocks of spatial reasoning itself!
Baggallini rolling tote your ultimate travel companion
Unlocking your potential understanding personality types carl jung
Unlocking the charm exploring the world of hello kitty aesthetic drawings